Exponential expressions involving the constant ‘e’ are crucial in various fields, including finance, science, and engineering. A quadratic calculator can be used to solve equations involving natural log. Understanding how to solve these exponential equations is essential for tackling real-world problems. This tutorial will help you practice solving quadratic and natural log equations. Mastery over such equations enables individuals to practice analyzing exponential growth or decay scenarios accurately using the natural log expression to answer problems.
Solving equations containing ‘e’ and logarithmic expressions allows us to model natural phenomena like population growth, radioactive decay, and compound interest. It’s important to practice solving these exponential expressions to find the answer. Understanding the behavior of electrical circuits and chemical reactions is vital. It also plays a crucial role in solving quadratic equations as a step to answer problems related to the sides.
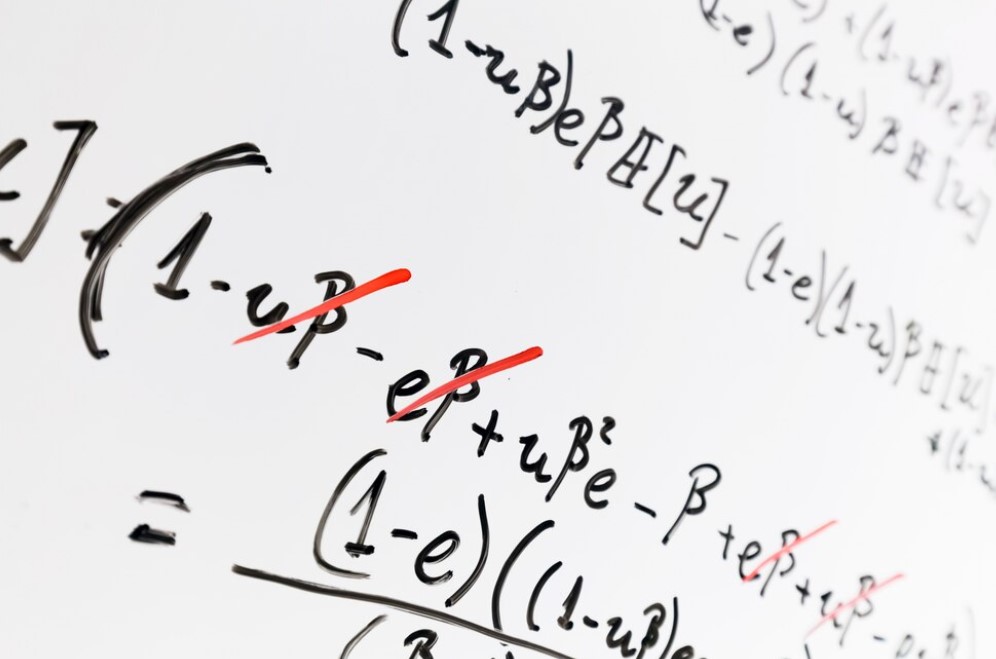
Brief History of the Constant ‘e’
The constant ‘e’ has a fascinating history in mathematics. The discovery of exponential equations can be traced back to the 17th century when Swiss mathematician Leonhard Euler first introduced it as the base for natural logarithms. This exponential expression has since become an essential part of understanding quadratic equations and finding their answer. The value of ‘e’ in exponential expressions and equations approximately equals 2.71828 and appears extensively in calculus and mathematical analysis. It is also used in quadratic equations and the natural log.
Euler’s work laid down the foundation for solving differential equations, making it an indispensable tool in physics, engineering, economics, and biology. It is a crucial step in understanding exponential expressions on both sides of the equation. Today, the quadratic equation, exponential expression, and exponential equation continue to hold immense significance across various scientific disciplines. The natural log also plays a crucial role in these areas.
Importance of Mastering Equations Involving ‘e’
Mastering exponential equation is a crucial step for students pursuing careers in STEM fields (science, technology, engineering & mathematics). It’s important to understand both sides of the equation. Proficiency in solving such equations enhances problem-solving skills by providing a deeper understanding of exponential functions and their applications. It is a crucial step to improving your skills and gaining a better understanding of both sides of the equation. Keep a log of your progress to track your improvement.
Understanding equations with ‘e’ empowers individuals to comprehend complex systems better by predicting future outcomes based on current conditions using exponential models. When working with these equations, it’s important to log each step and consider all sides of the equation. Proficiency in handling exponential expressions and logarithmic equations fosters critical thinking abilities necessary for analyzing data trends and making informed decisions.
Understanding the Constant ‘e’ in Mathematics
Understanding ‘e’
The constant ‘e’ is a fundamental number in mathematics, with a value approximately equal to 2.718. It plays a crucial role in exponential equations, exponential expressions, and logarithmic functions. Understanding its significance is a critical step in advanced mathematical concepts. The exponential equation plays a crucial role in various mathematical concepts and real-world applications. It is a fundamental step in solving the log function. One significant application of ‘e’ is in the calculation of compound interest, where it represents the base rate of growth over time in an exponential equation. It is a crucial step in solving logarithmic functions.
In finance, the first step in accurate predictions is to use ‘e’ and log, factoring in continuous compounding periods for investment growth. For instance, when calculating the future value of an investment that grows continuously at a certain annual interest rate, ‘e’ becomes essential in determining the final amount. This is a crucial step in the process and should be recorded in a log.
Significance and Examples
Moreover, ‘e’ also appears prominently in calculus and exponential functions as a key step in the mathematical log. When solving equations involving exponential growth or decay processes such as population growth or radioactive decay, understanding how to work with ‘e’ is paramount for keeping a log of the changes over time.
For example, consider a scenario where an initial quantity doubles every 3 hours due to exponential growth. In this scenario, the exponential growth can be tracked using a log. The equation representing this situation would involve the constant ‘e,’ showcasing its practical relevance beyond theoretical mathematics in a logarithmic log context.
Role in Mathematics
One can think of exponential functions as those that grow (or decay) at a rate proportional to their current size; they are prevalent across numerous scientific disciplines like physics and biology. Here’s where “the number e” comes into play – it’s deeply intertwined with these types of functions because any log function whose derivative is itself has something to do with e!
This connection between ‘e’, exponentials, derivatives, and logarithms forms one cornerstone behind its significance – it serves as the unique base for which both its function values (y-values) and their slopes (derivatives) remain identical! This property makes ‘e’-related calculations elegant since we don’t have to deal with awkward constants popping up during differentiation or integration processes. Additionally, keeping a log of these calculations can help in tracking the progress and making adjustments as needed.
Strategies for Isolating ‘e’ in Equations
Methods for Identifying ‘e’
When solving equations, it’s crucial to be able to log isolate specific variables. When dealing with the constant ‘log e’, there are several methods to identify its presence in an equation. One way is to look for expressions or terms that involve natural logarithms (ln). The natural logarithm function is directly related to the constant ‘e‘. Another method involves recognizing exponential functions where the base of the exponent is ‘e’. This can be done by analyzing the natural log of the function. For example, if an equation contains terms like e^x or e^(2x), then ‘e’ is present in the mathematical log.
It’s also important to understand that ‘e’, as a mathematical constant, can appear in various forms within equations. When working with mathematical equations, it’s crucial to keep a log of the different forms in which ‘e’ appears. It may not always be explicitly written as ‘e’, but rather embedded within different mathematical expressions, such as a logarithmic function.
Isolating ‘e’: Importance of Understanding Properties
Understanding the properties of ‘e’ when isolating it in equations is essential for accurate problem-solving. When working with logarithms, it’s crucial to understand the properties of ‘log’. One key property of ‘e’, which makes isolating it unique, is its relationship with natural logarithms. The inverse function of raising ‘e‘ to a power (exponential) is taking the natural logarithm.
Recognizing this relationship allows students to apply operations involving exponents and logs effectively when working with equations containing ‘e’.
Applying Logarithms to Eliminate ‘e’
Explanation of Using Logarithms
When dealing with equations containing ‘e’, one effective method for eliminating it is by using logarithms. By taking the natural logarithm (base e) on both sides of an equation, the exponent involving ‘e’ can be simplified and solved. This process allows us to transform the equation into a more manageable form without the presence of ‘e’. For instance, in the equation y = 3e^x, taking the natural logarithm on both sides results in ln(y) = ln(3) + x.
By applying this technique, we can isolate and solve for variables that were previously intertwined with ‘e’, making it easier to manipulate and analyze equations within various mathematical contexts.
Examples Demonstrating the Use of Logarithms
Let’s consider an example where we have an equation like 2e^(3x-1) = 10. To eliminate ‘e’, we apply natural logarithm on both sides:
ln(2e^(3x-1)) = ln(10)
Using properties of logarithms, this simplifies to:
ln(2) + (3x – 1)*ln(e) = ln(10)
Further simplifying gives us:
(3x – 1)*ln(e) = ln(10) – ln(2)
Since ln(e)=1, then:
3x – 1 = (ln(10)-ln(2))
Solving for x yields x=(ln(10)-ln(2)+1)/3
This demonstrates how applying logarithms helps remove ‘e’ from equations and facilitates solving for variables involved with exponential terms.
Benefits and Limitations
Using logarithms offers several benefits when eliminating ‘e’ from equations. It enables us to simplify complex exponential expressions involving ‘e‘, making them more amenable to algebraic manipulation. Through this method, we can effectively solve for unknowns that are linked with ‘e‘.
However, there are limitations associated with using logarithms in certain scenarios. For instance, when dealing with extremely large or small values involving ‘e‘, precision errors may arise due to limited computational resources or rounding issues during calculations.
Using Natural Logarithm Properties for Simplification
Overview of Natural Logarithm Properties
Natural logarithms, often denoted as ln, are essential when simplifying equations with ‘e’. The key property to remember is that the natural log of e equals 1. This means that if an equation contains ‘e’ and you take the natural log of both sides, the ‘e’ term will simplify to 1. Another important property is that when taking the natural log of a product, it can be expressed as the sum of the natural logs of each individual factor.
For instance:
ln(e) = 1
ln(ab) = ln(a) + ln(b)
Practical Examples Showcasing Application
Let’s consider an example where we have an equation with ‘e’, such as y = 3 * e^x. To eliminate ‘e’, we can apply natural logarithm properties by taking the natural log on both sides: ln(y) = ln(3 * e^x). According to the second property mentioned earlier, this simplifies into: ln(y) = ln(3) + x * ln(e). Since ln(e) equals 1, our equation becomes: ln(y) = x + ln(3).
Another practical example involves solving a trinomial equation containing ‘e’. If we have y = (2 * e^x)^2 – e^(2x), applying natural logarithm properties would entail breaking down each term using these properties before proceeding with further simplification.
Tips for Effective Utilization
When dealing with equations involving ‘e’, always keep in mind that applying natural logarithm properties allows for simplification without altering the fundamental nature of the original expression. Remembering and correctly utilizing these properties will help in effectively eliminating ‘e’ from various equations.
Practical Examples of Removing ‘e’ from Equations
Step-by-Step Walkthroughs
Let’s delve into specific equations to understand how to remove e. For instance, consider the equation y = 3e^x. To eliminate e, we can use the natural logarithm properties discussed earlier. By taking the natural logarithm of both sides, we get: ln(y) = ln(3e^x). Using the property that ln(a * b) = ln(a) + ln(b), we simplify it further as: ln(y) = ln(3) + lnx This allows us to isolate x by subtracting ln(3) from both sides: ln(y) – ln(3) = lnx
Now, we have successfully removed e from the equation and solved for x in terms of y.
Diverse Examples Illustrating Different Scenarios
Another example could be an exponential decay model represented by the equation: N(t) = N0 * e^(-rt). To get rid of e, you would apply a similar process. Take the natural logarithm on both sides and then solve for t using algebraic manipulation.
It’s crucial to note that each scenario might require a slightly different approach, but they all revolve around utilizing properties of natural logarithms to remove e effectively.
Emphasis on Understanding Thought Process
Understanding why and how certain steps are taken is essential when removing e from equations. This thought process involves recognizing which properties or rules of logarithms will help simplify or eliminate exponents involving e.
For example, in expressions like 2 * e^(2x), understanding that taking the natural log will allow you to bring down the exponent as a coefficient is key. It’s not just about following steps blindly but comprehending why those particular steps are applied in each situation.
Common Mistakes to Avoid When Dealing with ‘e’
Misidentification of ‘e’ in Equations
Equations containing ‘e’ can be tricky, and a common mistake is misidentifying the presence of ‘e’. It’s crucial to carefully scan through the equation and identify where the term appears. For instance, mistaking a variable for ‘e’ can lead to errors in calculations. Take, for example, the equation 3x = 5e – 2. Here, it’s essential to recognize that ‘e’ represents a constant value rather than a variable.
Another error related to identifying ‘e’ is overlooking its significance within an equation. Students often fail to grasp that ‘e’, Euler’s number, plays a unique role in mathematics due to its importance in exponential growth and decay equations.
Precision and Attention to Detail
Precision is key when handling equations involving ‘e’, as even minor errors can greatly impact the outcome of calculations. A common oversight is neglecting proper parentheses or brackets around terms containing ‘**‘ e’’. This can lead to inaccuracies during solving processes.
Attention to detail also comes into play when dealing with exponents that involve Euler’s number (‘e’) – failing to apply correct exponent rules might result in incorrect solutions. For example, mishandling exponents like e^2 or (3+4)e could lead to erroneous results if not approached meticulously.
Advanced Techniques for Complex Equations with ‘e’
Exploring Exponential Expressions
When dealing with complex equations involving the constant ‘e’, it’s essential to understand exponential expressions. These are mathematical expressions that involve the constant ‘e’ raised to a power, often represented as e^x, where x is any real number. In such cases, advanced techniques come into play to simplify and solve these intricate equations.
Understanding how exponential expressions interact within an equation can help in identifying when advanced techniques are necessary. For instance, when encountering equations containing terms like e^2x or 3e^(4x-1), it indicates the presence of exponential functions that require more than basic algebraic manipulation.
Applying advanced methods becomes crucial in scenarios where quadratic or higher-order polynomials intertwine with exponential expressions. This intersection calls for specialized techniques to untangle and solve these complex equations effectively.
Application through Examples
To grasp the application of advanced techniques in solving intricate equations involving ‘e’, consider an example:
Equation: 2e^(3x) – 7 = 0
Here, one would need to employ logarithmic functions or other sophisticated methods to isolate the variable x and solve for its value accurately. By applying these advanced strategies, mathematicians can effectively handle such challenging problems.
Another instance could involve decimal places within exponential terms: Equation: e^(0.5x) – 10 = 0
In this case, understanding how to manipulate exponents and work with decimal values becomes crucial in arriving at the correct solution.
Considerations for Complexity
Determining when to apply advanced techniques depends on the complexity of the equation at hand. If an equation involves multiple instances of ‘e’ coupled with various powers and coefficients, employing standard algebraic approaches might not suffice.
Considering factors such as multiple occurrences of ‘e’, varying powers as exponents, or intertwined quadratic elements guides mathematicians towards realizing when they should transition from conventional methodologies to more sophisticated ones.
Ultimately, recognizing key indicators within an equation signals when it’s time to pivot towards utilizing specialized strategies tailored for handling complex problems featuring exponential components.
Tools and Calculators to Assist with ‘e’ Equations
Online Resources
Several online resources can be incredibly helpful. Websites like Symbolab, Wolfram Alpha, and Desmos offer calculators specifically designed for handling equations with the constant ‘e’. These tools allow you to input your equation and receive step-by-step solutions, making it easier to grasp the process.
For example, Symbolab provides a user-friendly interface where you can enter your equation containing ‘e’ and instantly receive a breakdown of the solution. Similarly, Wolfram Alpha offers detailed explanations alongside its solutions, providing valuable insights into how the equation is manipulated.
Desmos stands out for its interactive graphing calculator that visualizes equations in real-time. This feature is particularly beneficial when dealing with exponential functions involving ‘e’, as it allows users to observe changes as they tweak different parameters within the equation.
Leveraging Technology
In addition to using dedicated calculators, leveraging technology while studying ‘e’ equations involves understanding how these tools arrive at their solutions. Rather than relying solely on the final answer provided by an online resource, take time to comprehend each step of the solution process.
By doing so, you’ll gain a deeper understanding of how ‘e’ operates within an equation and enhance your problem-solving skills in mathematics. It’s essential not only to obtain answers but also to grasp the underlying principles behind them.
Furthermore, consider using educational platforms such as Khan Academy or Coursera that offer courses on topics related to exponential functions and logarithms. These platforms provide video tutorials and practice exercises that reinforce learning through repetition and application.
Tips for Practicing and Mastering Equations Involving ‘e’
Consistent Practice
Consistent practice is key to mastering equations involving ‘e’. By regularly working through problems that contain the constant ‘e’, you can gradually build your understanding and confidence in solving such equations. Start with simple exercises, gradually progressing to more complex ones as you become more comfortable with the process. This steady approach allows you to internalize the techniques required to handle these equations effectively.
Engage in daily practice sessions, dedicating a specific amount of time each day to work on equations involving ‘e’. This regular commitment helps reinforce your problem-solving skills and ensures that you maintain a strong grasp of the necessary concepts. Consider setting aside 20-30 minutes every day for focused practice, allowing yourself ample opportunity to encounter various scenarios involving ‘e’.
Problem-Solving Approaches
Adopting effective problem-solving approaches is crucial when tackling equations containing ‘e’. Understanding the power rule step is essential for simplifying expressions involving exponential functions. By recognizing this fundamental technique, you can systematically address terms with ‘e’ and confidently navigate through different equation types.
When faced with an equation featuring ‘e’, break it down into smaller components and identify patterns within the expression. Utilize known mathematical rules, such as exponent properties and logarithmic functions, to manipulate terms containing ‘e’ effectively. For instance, when dealing with derivatives or integrals involving exponential functions, applying the power rule step enables you to differentiate or integrate these expressions methodically.
Incorporating Real-World Scenarios To deepen your comprehension of solving equations related to real-world applications incorporating ‘e‘, consider integrating practical scenarios into your practice sessions. For example, explore situations where exponential growth or decay occurs naturally in fields like finance, biology, or physics. By contextualizing mathematical concepts within tangible contexts, you develop a richer understanding of how ‘e‘ operates in real-life scenarios.
Analyze financial models that involve compound interest calculations based on continuous compounding using ‘e‘. Examine population growth models in biology that utilize ‘e‘ as a base for exponential growth rates over time periods. These real-world examples not only enhance your problem-solving abilities but also provide insight into how ‘** e **’ manifests itself across diverse disciplines.
Conclusion
You’ve now unlocked the secrets to conquering those tricky equations with ‘e’! By understanding the nature of the constant ‘e’ and implementing strategic techniques like logarithms and natural logarithm properties, you can confidently tackle equations involving ‘e’. Remember to steer clear of common mistakes and explore advanced methods for complex equations. Leverage available tools and calculators to streamline your problem-solving process. With consistent practice and determination, you’ll soon master the art of handling equations with ‘e’, paving the way for smoother mathematical journeys ahead.
Now, go forth and apply these newfound skills to crack those challenging equations with ‘e’ like a pro! Happy problem-solving!
Frequently Asked Questions
How can I isolate ‘e’ in an equation?
To isolate ‘e’ in an equation, you can use logarithms. By applying natural logarithms or log properties, you can eliminate ‘e’ and solve for the other variables. Understanding these strategies will help simplify equations involving ‘e’.
What are some common mistakes to avoid when dealing with ‘e’ in exponential expression and quadratic equations? One common mistake is not properly simplifying the squared terms. Another mistake is not knowing how to answer problem involving ‘e’.
One common mistake is forgetting to apply the proper logarithmic techniques to remove ‘e’. Misusing natural logarithm properties or neglecting to consider the constant nature of ‘e’ can lead to errors. Being mindful of these pitfalls enhances accuracy.
Are there tools or calculators available to assist with solving quadratic equations containing exponential expressions? These tools can help answer problems involving decimal places.
Yes, several online tools and calculators are specifically designed for handling equations involving constants like ‘e’. These resources can expedite complex calculations and provide step-by-step solutions, aiding your understanding of how to work with ‘e’.
Can you explain the significance of mastering quadratic equations involving ‘e’ and exponential expressions? How can a calculator help answer problems related to these equations?
Mastering such equations is akin to unlocking a powerful toolset in mathematics. It allows for more sophisticated problem-solving across various fields like calculus, finance, and physics. Proficiency with exponential functions enriches your quantitative skills.
How do advanced techniques help tackle complex quadratic equations featuring exponential expression? Use a calculator to answer problem.
Advanced techniques such as differentiation or integration play a crucial role in handling intricate equations containing ‘ e ’ . These methods enable a deeper understanding of the behavior of exponential functions and their interactions within complex mathematical models.



















